Deformation, Elasticity & Elastic Energy

Hooke's law

The force/load is directly proportional to the extension within the limit of proportionality

This is very important

Force = Spring contant * extension

F = kx

So this shows us that the extension changes with the load

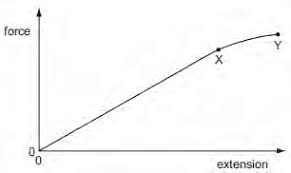
Where x is limit of proportionality and y is the elastic limit

However, when we plot a graph of the load and the (total) length of the wire, it's not directly proportional but, only linear

Spring constant

The force required per unit extension

F/x =k

The spring constant is unique for different springs, when you have different objects they have different spring constants. The spring constant measures how stiff a material is. So greater the spring constants, greater the stiffness

Definition

You will need to know some important terms:

• Hooke's law

Already explained



• Limit of proportionality

It is the maximum point where Hooke's law is obeyed and force is directly proportional to the load



• Plastic deformation

It is a deformation which when the load is removed, the object does not return to its original shape, it is permanently deformed



• Elastic deformation

The object returns to its original shape when the load is removed



• Elastic limit

The maximum point which when exceeded, the object is plastically deformed

Compressive/Tensile forces

We need to know what is the difference between compressive and tensile force

Compressive forces

Compression means squashing so the final lenght is actually less than the original length.

Tensile

Stretching, the final length is larger than the original length

Elastic potential energy

This is same as work done. However, we come to realise that the force changes with the extension, more force is required to extend it

So we take the average force ( (Max + Min)/2 )

Elastic potential energy = Average force * extension

E = ½Fx

The F is actual the maximum force because, the minimum force is zero - assuming that this is an extension-load graph which follows Hooke's law

Did you see it? This is the same as the area under the graph. So it is the same as finding the area of a triangle, that's why we times it by half!

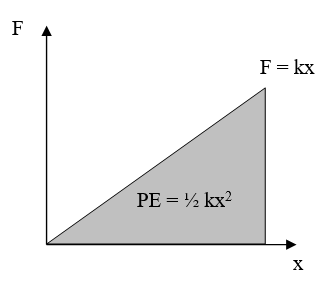
There is another way of expressing the same equation by replacing F with the spring constant

E = ½kx²

So half times the spring constant times the extension squared gives us the same value

Addition of spring constants

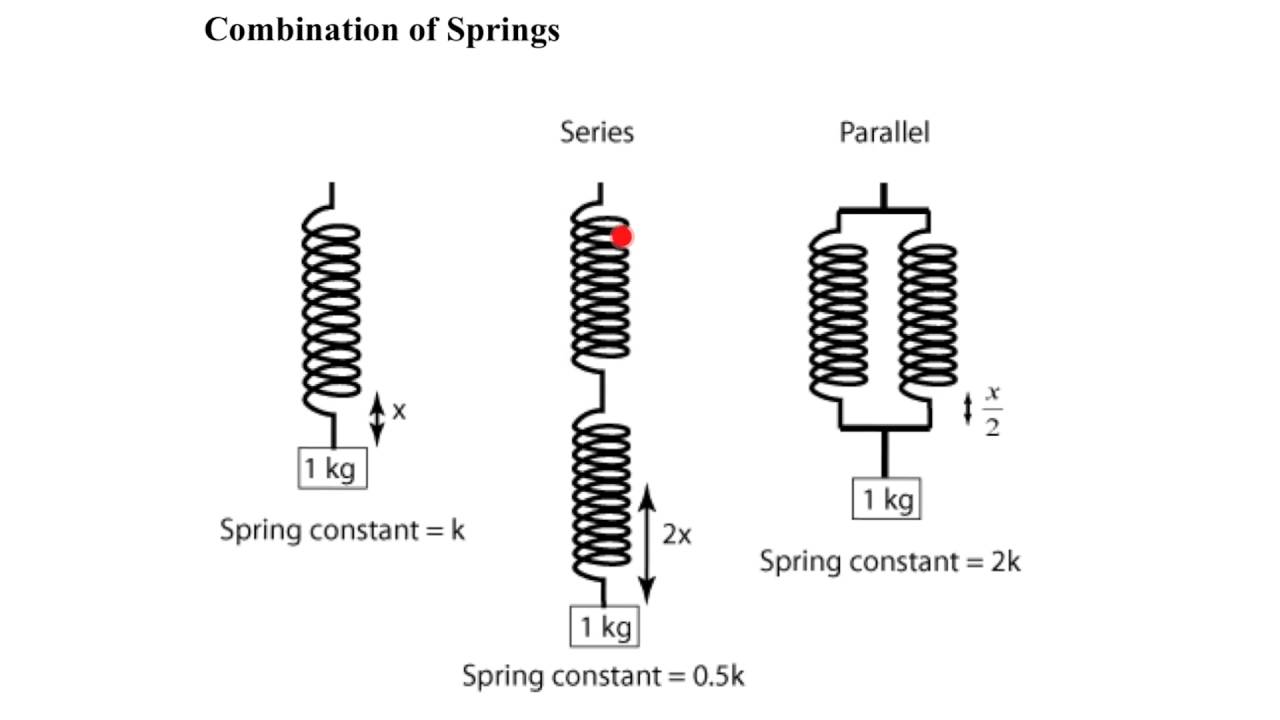
So the springs could be in different forms, all we need to do is think that the multiple springs acts as one huge spring

parallel spring combination

So when you place a load the force is spread out but, the extension is the same for each spring

To calculate it fast, just remember this

K_total = K₁ + K₂

A good way to remember is to think it as the opposite of adding the parallel circuit resistance

Proof

We will give a simple proof

As the force is distributed

F_total = F₁ + F₂

We can now write it in terms of kx only

K_totalx = K₁x + K₂x

The x is the same for all so we can just cancel it off and get this

K_total = K₁ + K₂

Series Spring combinations

1/K_total = 1/K₁ + 1/K₂

So after finding the sum of the 1/k of each springs you finally get 1/k_total. So you find the reciprocal to find the combined k

Remeber the Combined K would be less that the individual spring constants. Also remember it like this, it is the opposite of finding the sum of a Series circuit resistance

Proof

We know that the force exerted on each spring will be the same ( Think about tension of a rope, it's the same for all parts of the rope ). Each spring will have a different extension depending on their individual spring constants

Total extension = Extension₁ + Extension₂

X = F/K

F/K_total = F/K₁ + F/K₂

So as the force is common, we could remove the F out

1/K_total = 1/K₁ + 1/K₂

Remember these proofs are not neccesary but, good to remember by any chance you forget! If you don't understand there will be a video on this down below

Here is an example - each spring has a spring constant of K

Young modulus ( E )

So we have seen above, that different thicknesses and lengths of springs are used and so we get different spring constants even if the same material is used. Think about it!

If I have a very fat spring made out of iron and a thin spring made out of iron, which is easier to pull?

So there must be a standard way of measuring the extension of a material for a given force and for a unit length and cross-sectional area. This is known as Young modulus.

It is the ratio between stress and strain

Young modulus = Stress/Strain

Let's see first what's stress and strain

Stress

The force acting on per unit cross sectional area of a wire

Stress = Force/Cross-Sectional area

Stress = F/A

As you can see, this is very similar to pressure and it shows us how much load the wire handles per unit cross-sectional area

So it has the same units as pressure : Pascals

SI units: Pa or Nm^-2

I have avoided the Greek symbols for these because, it is not important

Strain

The extension per unit original length of the wire

Strain = Extension / Length

Strain = x/l

So when we apply a force to a wire, obviously it will act like a spring. And it will extend(very little). So the ratio between the extension and the original wire is called strain

It has no units as the units cancel off. It can be written off as a ratio or a percentage. However be aware that in MCQs and past papers, they sometimes give it in a percentage. So convert it first to a ratio ( by dividing by 100 ) before using it in your calculations

So now we can get Young Modulus by dividing it by strain

Young Modulus = (F/A)/(x/l)

This is same as:

Young modulus = Fl/Ax

So what does this show:

It shows the force required to extend a wire of (original) length of 1m and cross sectional area of 1m² by 1m

So the units of Young Modulus is the same as stress as strain has no units

SI units: Pa or Nm^-2

However exam question usually ask what is the estimate for the young modulus of a wire and it's usually in GigaPascal/GPa or MPa

Also remember this:

Young Modulus value is the Same for the same type of material

So even if the area or the length changes, the young modulus of a steel wire will always be the same ( at a constant temperature ) - Only the extension and the force required will change.

Stress and Strain Graphs

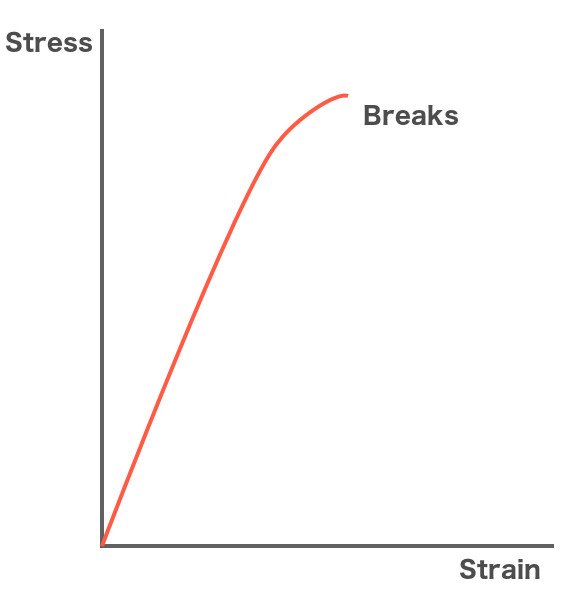
Very similar to the force-extension graph, it has a limit of proportionality.

However, the gradient represents Young Modulus

The gradient of stress and strain graph = Young modulus

The area under the graph measures the Elastic potential energy per unit volume

This is very important also:

Elastic energy per unit volume

Energy per unit Volume = ½Stress*Strain

We take half as we use the maximum stress and so we multiply by ½ to get the average. This is only used when the graph is a straight line.

It is the same as Force because, stress also increases linearly , so we get the maximum stress and divide it by 2

There are more graphs on stress and strain you need to know

Ultimate Tensile Strength

It is the maximum stress a object can handle before it breaks

After the peak the stress decreases and the wire breaks. This is at the fracture point but, this is not needed.

Ductile or Brittle

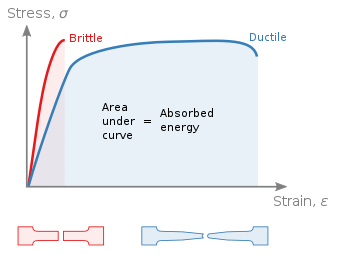
Both brittle and ductile materials follow Hooke's law, however brittle items break easily, whereas ductile material extends and plastically deforms

Work done by Springs/Wires

When we extend the spring, Elastic potential energy is stored in the spring and that's shown by the area of the force-extension graph. This is called Work done on the spring

However, when the spring is released, Work is done by the spring and if it is elastically deformed, the object comes to its original shape.

So if we put it on a graph, it will be no different as the work done on the spring is the same as the work done by the spring so the areas overlap perfectly

However, if we extend it beyond the elastic limit, the object is plastically deformed and it will keep it's new shape, so energy will be transfered out in the form of heat and so work done by the spring will be less than the work done on the spring. The difference would be the energy lost in the form of heat

Energy loss = Work done on spring - Work done by the spring

Measuring Young Modulus

We know what's included in Young Modulus

Young modulus = Fl/Ax

So we need to find each quantity

• Force/load

Using different standard mass such as 100g and 50g as the force

• Length

You can use a meter ruler to measure the original length of the wire. You need to measure the length from the start of the wire, (attached to a G clamp) to the point or mark/pointer on the wire

• Extension

Using a travelling microscope to measure the change of length showed by the pointer on the wire

• Cross sectional area

Using a micrometer screw guage to find the diameter and the area

Things to remember!




Plastic deformation graph



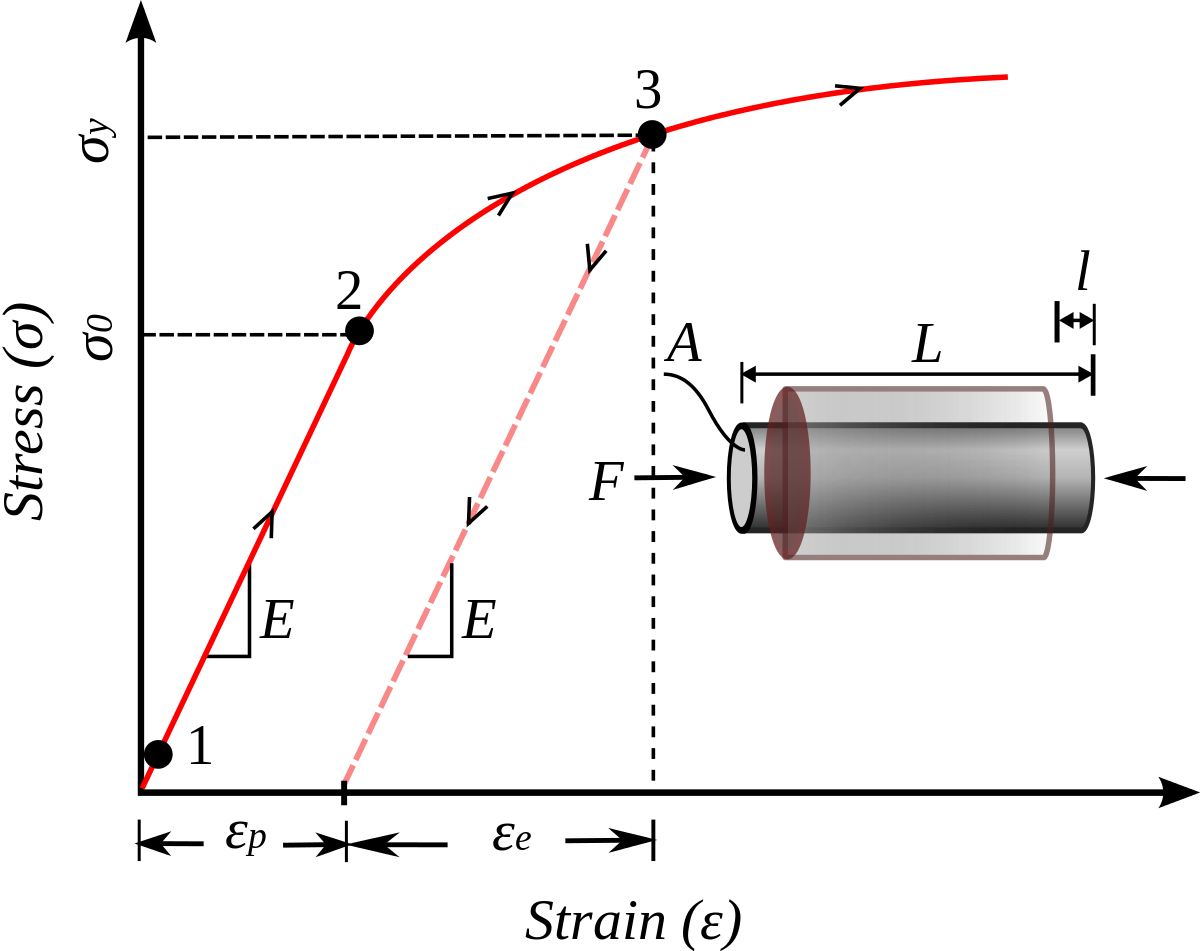
So this shows that when the wire is extended more than the elastic limit. The wire follows a new Spring constant and it decreases in a straight line. This should be remembered.




Work Lost/Wasted



Some things needed to be noted here. When we are extending the wire and removing the load, sometimes the stress-strain graph line does not overlap(non hysteresis). And so the difference in work done on the spring and the work done by the spring is the lost energy.

This is the shaded area but, as this is the stress-strain graph, this shows the heat loss per unit volume.

Moreover, you need to remember that the heat loss is for both extending and releasing the wire ( one cycle ) and not just for extending the wire.

Lastly, keep this in mind too!

You should use thin wires instead of thick ones as thin wires stretch more

Use a large length of wire




Back Next



Recommended

These are things you might like. Clicking these ads can help us improve our free services in the future...




End of Chapter Questions

Collection of Topic Questions to understand the topic more.


Try out




End of Chapter Videos

Collection of Videos to Support Your Understanding.

Remember these videos are handpicked by me and I feel these are the best ones out there. But I constantly update this list for each chapter. The Youtubers are more than welcome to contact me.

Also, don't forget to Subscribe to our Youtube channel - MrWik


Watch